7.1.2 The Coincidence Site Lattice

If we look at the infinity of possible orientations of two grains relative to each other, we will find some special orientations. In two dimensions this is easy to see if we rotate two lattices on top of each other.

You can watch what will happen for a hexagonal lattice lattice by activating the link.

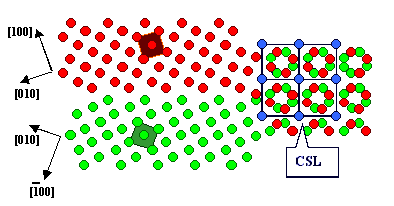
A so-called Moirée pattern develops, and for certain angles some lattice points of lattice 1 coincide exactly with some lattice points of lattice 2. A kind of superstructure, a coincidence site lattice (CSL), develops. A question comes to mind: Do these special coincidence orientations and the related CSL have any significance for grain boundaries?

Lets look at our paragon of grain boundaries, the twin boundary:

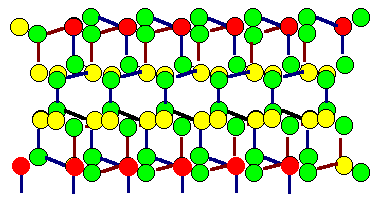
Shown are the two grains of the preceding twin boundary, but superimposed. Coinciding atoms (in the projection) are marked red. However, this might be coincidental (excuse the pun), because the atoms in this drawing are not all in the drawing plane. Note that it is not relevant if the boundary itself is coherent or not - only the orientation of the grains counts.

And once more, note that the lattice is not the crystal! We are looking for coinciding lattice points - not for coinciding atom positions (but this may be almost the same thing with simple crystals).

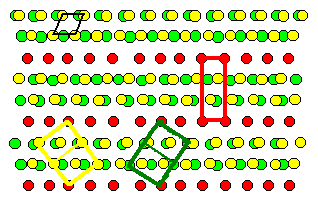
So in this picture the same situation is shown for the fcc lattice belonging to the grain boundary. Again, coinciding lattice points are marked red and a (two-dimensional) elementary cell of the CSL is also shown in red. The two (three-dimensional) elementary cells of the fcc lattices are also indicated.

It is definite from this picture that the the twin boundary belongs to the class of boundaries with a coincidence relation between the two lattices involved.

From the animation in the link above it was clear that many coincidence relations exist for two identical two-dimensional lattices. In order to be able to extend the CSL consideration to three dimensions and to generalize it, we have to classify the various possibilities. We do that by the following definition:

Definition:
The relation between the number of lattice points in the unit cell of a CSL and the number of lattice points in a unit cell of the generating lattice is called Σ (Sigma); it is the unit cell volume of the CSL in units of the unit cell volume of the elementary cells of the crystals.

A given Σ specifies the relation between the two grains unambiguously - although this is not easy to see for, let's say, two orthorhombic or even triclinic lattices.

If we look at the twin boundary situation above, we see that Σ_twin = 3 (you must relate the two-dimensional lattices her; one is pointed above out in black!). For the three-dimensional case we still obtain Σ = 3 for the twin boundary, so we will call twin boundaries from now on: S3 boundaries.

A Σ1 boundary thus would denote a perfect (or nearly perfect) crystal; i.e. no boundary at all. However. boundaries relatively close to the Σ1 orientation are all boundaries with only small misorientations called "small-angle grain boundaries" - and they will be subsumed under the term Σ1 boundaries for reason explained shortly.

Since the numerical value of Σ is always odd, the twin boundary is the grain boundary with the most special coincidence orientation there is, i.e. with the largest number of coinciding lattice points.

Next in line would be the Σ5 relation defining the Σ5 boundary. It is (for the two-dimensional case) most easily seen by rotating two square lattices on top of each other.

This also looks like a pretty "fitting" kind of boundary, i.e. a low energy configuration.

A suspicion arises: Could it be that grain boundaries between grains in a CSL orientation, especially if the Σ values are low, have particularly small grain boundary energies?

The answer is: Yes, but... . And the "but" includes several problems:

Most important: How do we get an answer? Calculating grain boundary energies is still very hard to do from first principles (Remember, that we can't calculate melting points either, even though its all in the bonds). First principles means that you get the exact positions of the atoms (i.e. the atomic structure of the boundary and the energy). Even if you guess at the positions (which looks pretty easy for a coherent twin boundary, but your guess would still be wrong in many cases because of so-called "rigid body translations"), it is hard to calculate reliable energies.

So we are left with experiments. This involves other problems:

How do you measure grain boundary energies?

How do you get the orientation relationship?

How do you account for the part of the energy that comes from the habit plane of the boundary - after all, a coherent twin (habit plane = {111}) has a much smaller energy than an incoherent one?

Getting experimental results appears to be rather difficult or at least rather time consuming - and so it is!

Nevertheless, results have been obtained and, yes, low Σ boundaries tend to have lower energies than average.

However, the energy does not correlate in an easy way with Σ; it does not e.g. increase monotonously with increasing Σ. There might be some Σ values with especially low energy values, whereas others are not very special if compared to a random orientation.

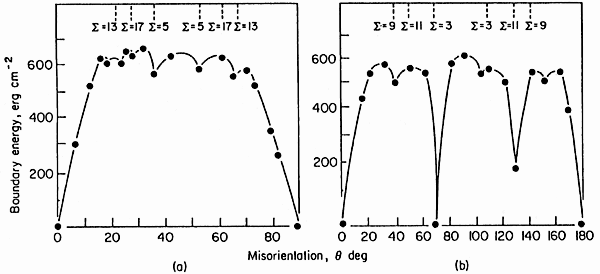
The result of (simple) calculations for special cubic geometries are shown in the picture:

Shown is the calculated (0^oK) energy for symmetric tilt boundaries in Al produced by rotating around a <100> axis (left) or a <110> axis (right). We see that the energies are lower, indeed, in low Σ orientations, but that it is hard to assign precise numbers or trends. Identical Σ values with different energies correspond to identical grain orientation relationships, but different habit planes of the grain boundary.

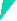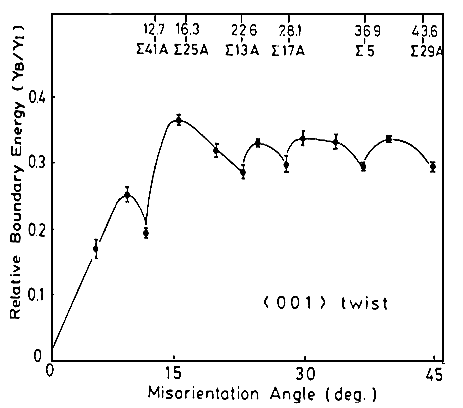
The next figure shows grain boundary energies for twist boundaries in Cu that were actually measured by Miura et al. in 1990 (with an elegant and ingenious, but still quite tedious method).

Clearly, some Σ boundaries have low energies, but not necessarily all.

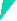Nevertheless, in practice, you tend to find low Σ boundaries, because (probably) all low energy grain boundaries are boundaries with a defined Σ value. And these boundaries may have special properties in different contexts, too.

The link shows the critical current density (at which the superconducting state will be destroyed) in the high-temperature superconductor YBa₂Cu₃O₇ with intentionally introduced grain boundaries of various orientations and HRTEM image of one (facetted) boundary. It is clearly seen that the critical current density has a pronounced maxima which corresponds to a low Σ orientation in this (Perovskite type) lattice.

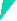However, despite this or other direct evidence for the special role of low Σ boundaries, the most clear indication that low Σ boundaries are preferred comes from innumerable observations of a different nature altogether - the observation that grain boundaries very often contain secondary defects with a specific role: They correct for small deviations from a special low Σ orientation.

In other words: Low Σ orientations must be preferred, because otherwise the crystal would not "spend" some energy to create defects to compensate for deviations.

If we accept that rule, we also have an immediate rule for preferred habit planes of the boundary:

Obviously, the best match can be made if as many CSL points as possible are contained in the plane of the boundary. This simply means:

Preferred grain boundary planes are the closest packed planes of the corresponding CSL lattice.

We will look at those grain boundary defects in the next sub-chapter.